搜索结果: 1-15 共查到“动力系统”相关记录18条 . 查询时间(1.656 秒)

Academy of Mathematics and Systems Science, CAS Colloquia & Seminars:Convergence of the Planewave Approximations for Incommensurate Systems
不可测系统 平面波逼近 收敛性
2023/12/11
带有固执个体的级联观点动力学的同步研究
固执个体 级联观点 动力学 同步研究
2023/12/13
中国科学院合肥物质科学研究院专利:一种电子驻车制动系统的电源模块
中国科学院合肥物质科学研究院 专利 电子驻车 制动系统 电源模块
2023/9/14

北京大学110周年庆·数学学人 | 文兰:优美深邃的微分动力系统(图)
文兰 北大 数学 微分动力系统
2023/6/16
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars: Derivation of the Vlasov equation from quantum many-body Fermionic systems with singular interaction
奇异相互作用 量子多体 费米子系统 弗拉索夫方程
2023/5/12
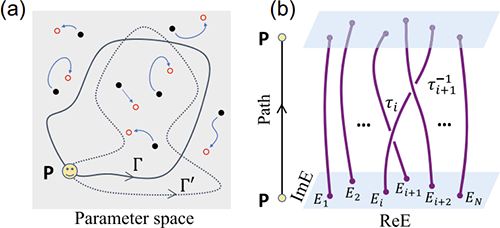
非厄米多带系统中例外简并的非阿贝尔拓扑(图)
非厄米多带系统 简并 非阿贝尔拓扑
2023/4/24
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars:Ergodic Theory and Semisimple Groups
遍历理论 半简单群 马古利斯超刚性定理
2023/4/13
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars:A deformation of Borel equivariant homotopy
博雷尔等变 同伦变形 R-动力稳定
2023/4/18
质点-弹簧-惯容系统的特征值反问题(李婵颖、谢奇达)
质点-弹簧-惯容系统 特征值 反问题
2023/2/22
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars: A decoupled, linear, and unconditionally energy stable finite element method for a two-phase ferrohydrodynamics model
两相铁流体 动力学模型 能量稳定 有限元方法
2023/4/28

中国科学院数学物理学部龙以明院士(图)
中国科学院数学物理学部 哈密尔顿动力系统 龙以明 院士
2022/9/8

中国科学院数学物理学部郭柏灵院士(图)
中国科学院数学物理学部 非线性发展方程 无穷维动力系统 郭柏灵 院士
2022/9/8
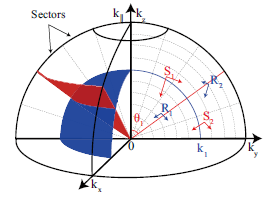
螺旋旋转湍流中能量/螺旋度联合输运机制研究新进展(图)
螺旋旋转湍流 能量/螺旋度 联合输运机制
2023/1/7


